What Is The Limit Process

DERIVATIVES USING THE LIMIT DEFINITION

The following problems require the utilise of the limit definition of a derivative, which is given by

$tex2html_wrap_inline338$ .

They range in difficulty from like shooting fish in a barrel to somewhat challenging. If y'all are going to attempt these problems earlier looking at the solutions, you can avoid common mistakes by making proper apply of functional note and conscientious apply of basic algebra. Keep in mind that the goal (in most cases) of these types of problems is to be able to divide out the $tex2html_wrap_inline29$ term and then that the indeterminant grade $tex2html_wrap_inline31$ of the expression can be circumvented and the limit can be calculated.

Trouble ane : Apply the limit definition to compute the derivative, f'(x), for
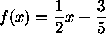$tex2html_wrap_inline35$ .

Click Hither to see a detailed solution to problem 1.
Problem ii : Utilise the limit definition to compute the derivative, f'(10), for
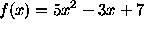$tex2html_wrap_inline39$ .

Click HERE to run into a detailed solution to problem 2.
Trouble 3 : Use the limit definition to compute the derivative, f'(x), for
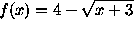$tex2html_wrap_inline43$ .

Click Hither to see a detailed solution to problem 3.
PROBLEM 4 : Utilise the limit definition to compute the derivative, f'(x), for
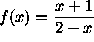$tex2html_wrap_inline47$ .

Click Here to run into a detailed solution to problem 4.
PROBLEM v : Use the limit definition to compute the derivative, f'(x), for
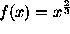$tex2html_wrap_inline51$ .

This problem may be more difficult than it first appears.

Click HERE to run across a detailed solution to problem v.
Trouble 6 : Employ the limit definition to compute the derivative, f'(10), for
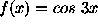$tex2html_wrap_inline55$ .

Click Here to see a detailed solution to problem 6.
PROBLEM vii : Use the limit definition to compute the derivative, f'(ten), for
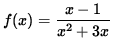$f(x) = \displaystyle { x - 1 \over x^2 + 3x }$ .

Click HERE to encounter a detailed solution to problem seven.
Trouble 8 : Use the limit definition to compute the derivative, f'(10), for
$f(x) = \sqrt{ x^3 - x }$ .

Click HERE to see a detailed solution to problem 8.
Problem nine : Assume that
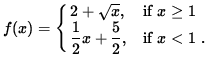
$f(ten) = \cases{ 2 + \sqrt{ x }, & if $\space ten \ge 1 $\infinite \cr \displaystyle{ 1 \over 2 } 10 + \displaystyle{ v \over 2 } , & if $ x < 1 $\space . \cr }$

Show that f is differentiable at ten=1, i.e., use the limit definition of the derivative to compute f'(1) .

Click HERE to see a detailed solution to problem 9.
PROBLEM 10 : Assume that
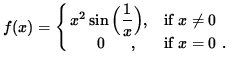
$f(x) = \cases{ x^2 \sin \Big( \displaystyle{ 1 \over x } \Big), & if $\space x \ne 0 $\space \cr \ \ \ \ \ 0 \ \ \ \ \ , & if $ x = 0 $\space . \cr }$

Show that f is differentiable at ten=0, i.east., use the limit definition of the derivative to compute f'(0) .

Click HERE to see a detailed solution to problem 10.
PROBLEM xi : Use the limit definition to compute the derivative, f'(10), for
f(x) = | x ^two - 3x | .

Click HERE to see a detailed solution to problem 11.
Trouble 12 : Assume that
$f(ten) = \cases{ \displaystyle{ 1\over 4 }10^3 - \displaystyle{1 \over two } x^2, &... ...$\space \cr \displaystyle{ -6x-half-dozen \over 10^2+two } , & if $ 10 < 2 $\space . \cr }$

Determine if f is differentiable at ten=ii, i.e., make up one's mind if f'(2) exists.

Click Here to run across a detailed solution to trouble 12.